One of a triangles points of concurrency. It turns out that all three altitudes always intersect at the same point - the so-called orthocenter of the triangle.

Point Of Concurrency In A Triangle Definitions Facts And Solved Examples Cuemath
Want to see the video.
. Then the point is equidistant from the endpoints of the segment. Any point that is on the perpendicular bisector of a segment is equidistant from the endpoints of that segment. A consequence of this finding is that decreasing the size of a median to 20 feet 61 m from 30 feet 91 m to add lanes to a.
A centroid divides the median in the ratio 2. CC Geometry 10-1-4 CW. Find the centroid of the triangle the coordinates of whose vertices are given by Ax1 y1 Bx2 y2 and Cx3 y3 respectively.
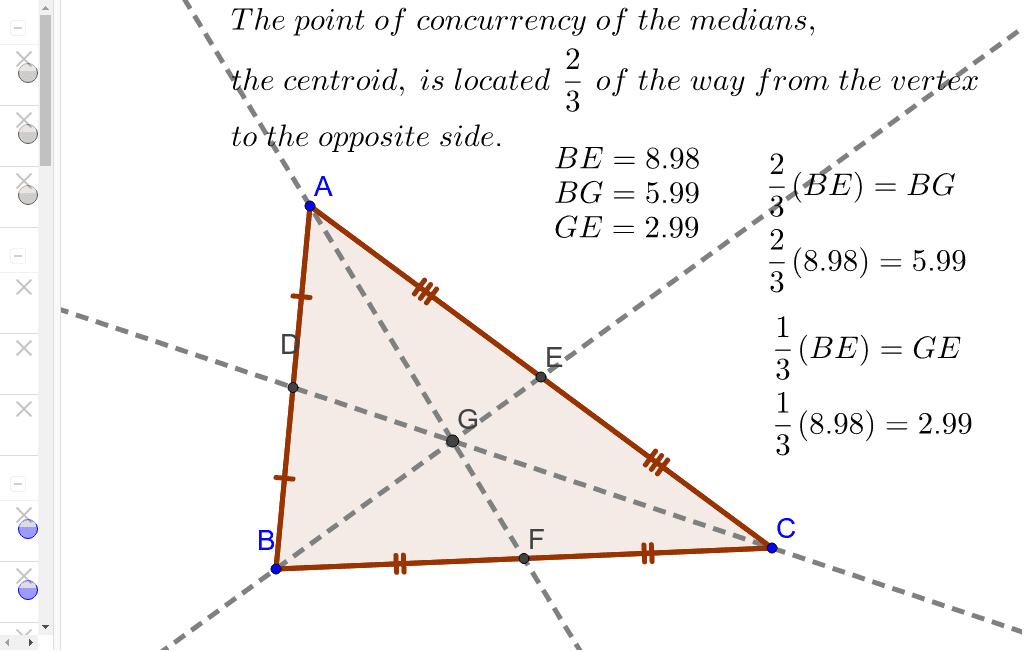
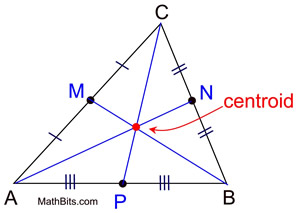
The line segments of medians join vertex to the midpoint of the opposite side. The altitudes of a triangle meet at the orthocenter. The point of concurrency is known as the centroid of a triangle.
Adjust the figure above and create a triangle. Centroid to midpoint 21. Please Use Our Service If Youre.
Concurrency of Medians Theorem Concurrency of Altitudes Theorem Inequalities Comparison Property of Inequality Corollary to the Triangle Exterior Angle Theorem Theorem 5-10 Theorem 5-11 Theorem 5-12 Triangle Inequality Theorem Hinge Theorem Converse of the Hinge Theorem. A median is the line joining the mid-points of the sides and the opposite vertices. The point of intersection of the medians of a triangle is known as centroid.
The study found that medians without barriers should be constructed more than 30 feet 91 m wide in order to have any effect on safety and that safety benefits of wider medians continue to increase to a width of 60 to 80 feet 183 to 244 m. A centroid divides the median in the ratio 21. We provide solutions to students.
View solution In the adjoining figure find x y z w. Before understanding the point of concurrency let us discuss the medians of a triangle. CC Geometry 10-1-4 Intro Activity and Resource Pagepdf We will go over the proof 10-42 when we meet tomorrow for class Resource Page Key.
Wishing for a unique insight into a subject matter for your subsequent individual research. Each median of a triangle divides the triangle into two smaller triangles that have equal areas. The circumcenter of a triangle is equidistant from the midpoints of each side of the triangle.
How To Find the Altitude of a Triangle. Learn the point of concurrency definition and the four. Every triangle has exactly three medians.
Label the point of intersection of AB and XY as M the midpoint of AB. 5 minutes All levels English. Three altitudes can be drawn for any one triangle.
The orthocenter can be inside. View solution View more. In the given figure AD BF and CE are medians of a triangle ABC and O is a point of concurrency of medians.
If the triangle is obtuse it will be outside. The centroid is the center of mass of a triangle and it divides each median into a ratio of 21 vertex to centroid. Points of Concurrency and Pyramids Worksheet Key.
A median of a. 5 minutes All levels English. A centroid is the point of intersection of the medians of the triangle.
Learn the formula for how to find the altitude of a triangle and calculate altitudes for equilateral isosceles and right triangles. If AD 6 cm then OD is equal to. It is much better at detecting distributional differences when the sample medians are far apart than it is at detecting when the tails are different but the main mass of the distributions is around the same values.
A centroid is also known as the centre of gravity. From the given figure three medians of a triangle meet at a centroid G. To make this happen the altitude lines have to be extended so they cross.
Concurrency of the three medians of a triangle. Concurrency of the three altitudes of a triangle. So more formally suppose are n independent and identically distributed observations of a continuous value.
All three medians meet at a single point concurrent. View solution Find the lengths of the medians of a A B C having vertices at A 0 1 B 2 1 and C 0 3 Medium. Medians are the line segments that are drawn from the vertex to the mid-point of the opposite side of the vertex.
1014 We learned that a line tangent to a circle is perpendicular to the radius of the circle drawn to the point of tangency. The empirical cumulative distribution function is. Properties of the Centroid of Triangle.
The orthocenter is not always inside the triangle.
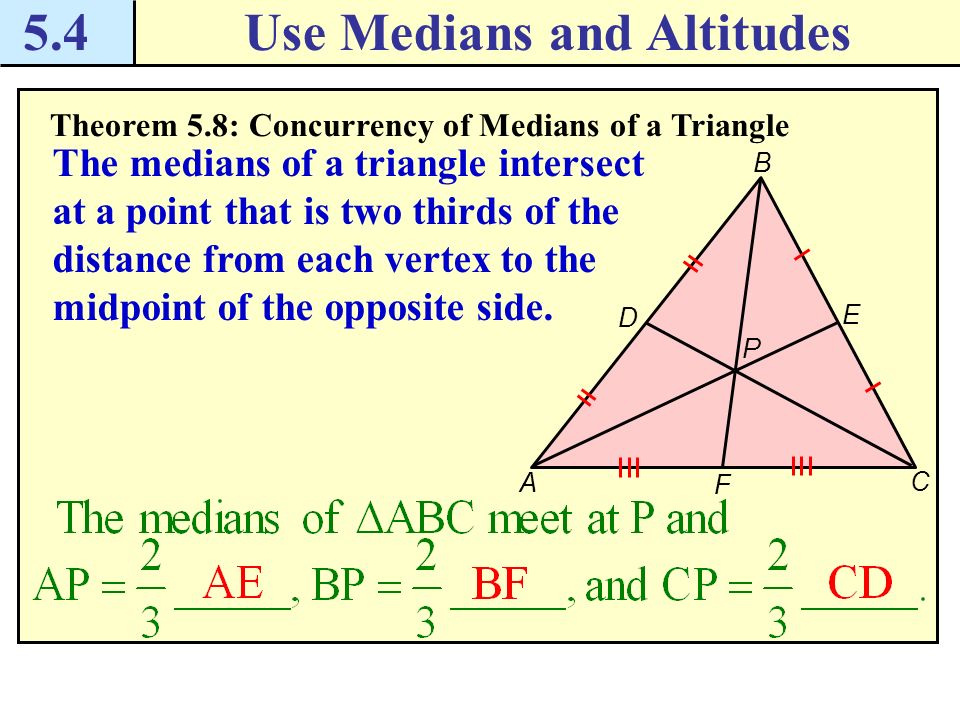
5 4use Medians And Altitudes Theorem 5 8 Concurrency Of Medians Of A Triangle The Medians Of A Triangle Intersect At A Point That Is Two Thirds Of The Ppt Download
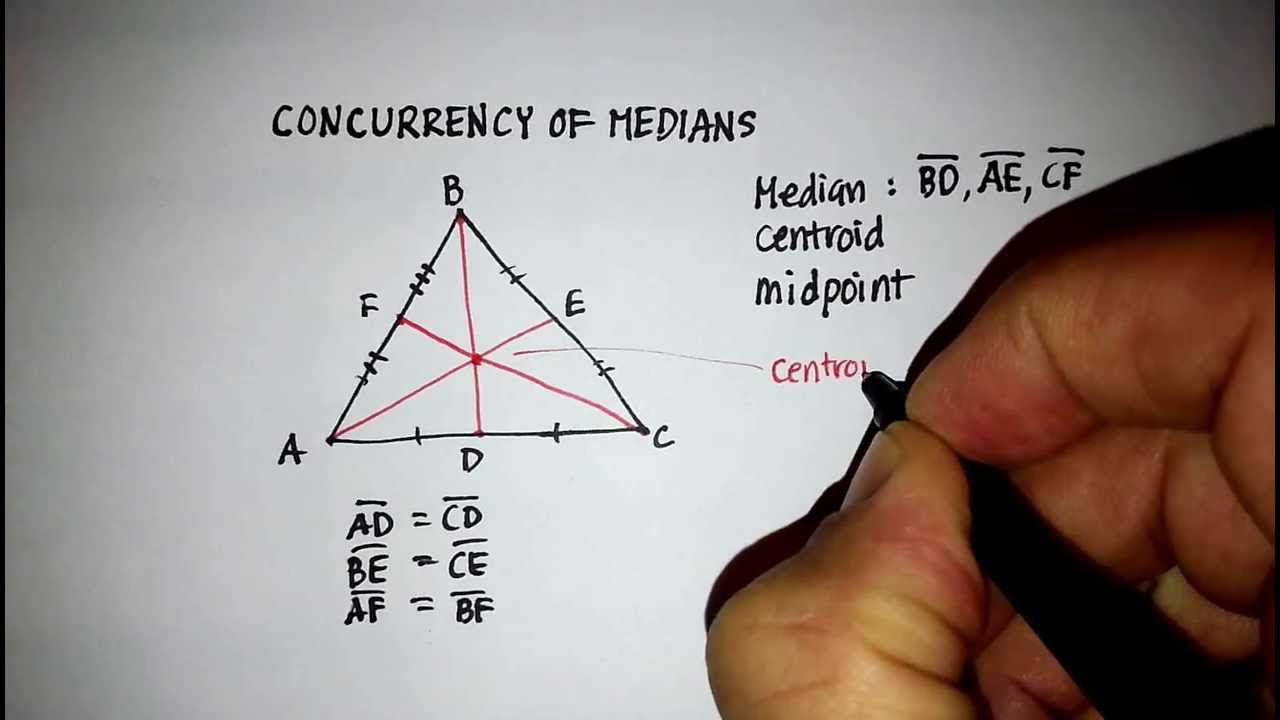
Triangles Concurrency Of Medians Centroid Youtube
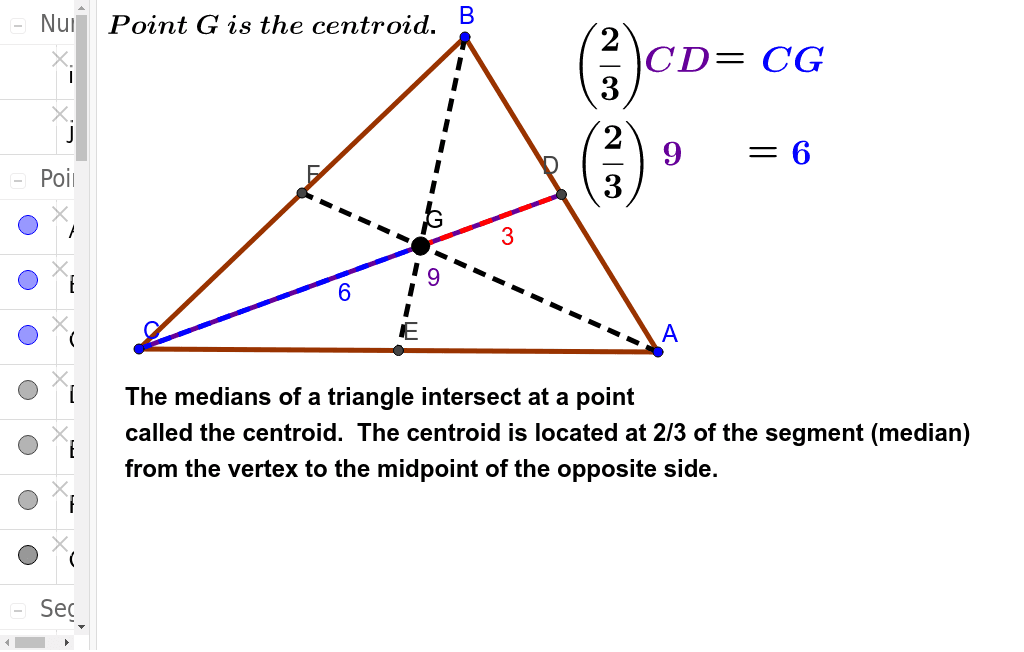
Point Of Concurrency Of Medians Centroid Geogebra
Emat6680 Gif Assignment 4 Concurrence Of Medians By Jenny Johnson Medians A Median Of A Triangle Is The Segment From A Vertex To The Midpoint Of The Opposite Side Each Triangle Will Have Three Medians One Segment From Each Of The Three

Point Of Concurrency In A Triangle Definitions Facts And Solved Examples Cuemath

Point Of Concurrency Of Medians Centroid Geogebra
0 comments
Post a Comment